Question

Can someone please answer this for me i cant figure it out.

Answer 1

x^(2/3)

Explanation:

(x^a.x^b)^c = x^[c*(a+b)]

using the above eqn, u can simplify the given expression to

x^[1/3*(4/3+2/3)]

=x^[1/3*(6/3)]

=x^(2/3)

ans is the 2nd choice

Answer 2

`\displaystyle x^{(2)/(3)}`

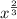
Explanation:

The rules of exponents tell you ...

... (a^b)(a^c) = a^(b+c) . . . . . . applies inside parentheses

... (a^b)^c = a^(b·c) . . . . . . . . applies to the overall expression

The Order of Operations tells you to evaluate inside parentheses first. Doing that, you have ...

... x^(4/3)·x^(2/3) = x^((4+2)/3) = x^2

Now, you have ...

... (x^2)^(1/3)

and the rule of exponents tells you to multiply the exponents.

... = x^(2·1/3) = x^(2/3)