Answer : The value of constant of variation is, 540
Step-by-step explanation :
As we know that,

That means,
d = s × t
Here,
's' is constant of variation.
Given:
d = distance = 810 km
t = time = 1.5 hr
Now put the values in the above expression, we get:
d = s × t
810 km = s × 1.5 hr
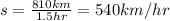
Thus, the value of constant of variation is, 540