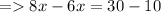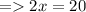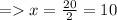Question

Given m|n, find the value of x.
(8x+10)
mm
(6x+30)
>
Answer:

Answer 1

The value of X is 10.

Explanation:

solution

(8x+10)=(6x+30) (alternate angle)

8x-6x=30-10

2x=20

X=20/2

X=10

Answer 2

x = 10

Explanation:

When any 2 lines are parallel to each other and a transversal intersects both the lines , then THE INTERIOR ALTERNATIVE ANGLES ARE ALWAYS EQUAL.

According to the question , m || n & t is transversal. So, the interior alternative angles will be equal.

`= > 8x + 10 = 6x + 30`

`= > 8x - 6x = 30 - 10`

`= > 2x = 20`

`= > x = (20)/(2) = 10`