Question

According to the fundamental theorem of algebra, how many zeros does the polynomial below have?

Answer 1

2? i've never learned this but i just tried to teach myself lol sorry if its wrong i tried

Explanation:

Answer 2

The given polynomial has 4 roots.

( may not be all distinct)

Explanation:

Fundamental Theorem of Algebra --

It states that every non-constant polynomial of degree n has exactly n roots counting multiplicity.

i.e. the roots may repeat.

i.e. the number of roots of a non-constant polynomial of degree n has atmost n distinct roots.

We are given a polynomial as:

`f(x)=x^4+5x^3+10x^2+20x+24`

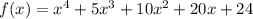
Clearly the polynomial is of degree 4.

This means that the roots of this polynomial will be: 4

( not all roots may be distinct)