Question

A certain forest covers an area of 1700 km^2 . Suppose that each year this area decreases by 6.5%.What will the area be after 5 years?

Answer 1

12476.4 km^2

Explanation:

If we have an area of 1700 km^2 and it decreases at a rate of 6.5% a year then, 6.5% is 6.5÷100=0.065 in decimals. Since the amount of forest area is decreasing from the original 100% by 6% each year then 1-0.06=0.94 or 94% of the forest is left every year and so:

`F_(final)=F_(initial)(1+r)^t`

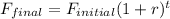
Where the initial forest is 1700 km^2 , the rate is r=-0.06 (negative since it's decreasing) and t is the time so t=5.

By plugging in those values we have:

`F_(final)=1700(1-0.06)^5=1700(0.94)^5=12476.4km^2`

The amount of forest left after 5 years is 12476.4 km^2.