Answer:
Given the function:
f(x) = the quantity of x squared plus 3x minus 4 , all over x plus 4
i,e
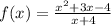
or

or
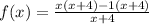
or

⇒y= f(x) = x -1 ......[1]
Find the x-intercepts and y-intercepts for the equation [1] ;
y-intercepts defined as the graph crosses the y-axis;
substitute x =0 to solve for y;
y = 0-1 = -1
y = -1
x-intercepts defined as the graph crosses the x-axis;
substitute y =0 to solve for x;
0 = x-1
or
x = 1
Plot these points (0 , -1) and (1, 0) on the graph for the given function as shown below.