Question

In order for the parallelogram to be a
rhombus, x = [?].
(7x-44)°
(11x-76)

Answer 1

`\boxed{\sf{x=8}}`

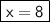
Explanation:

These two bisected angles must be equal for the parallelogram to be a rhombus.

11x-76=7x-44

Isolate the term of x, from one side of the equation.

First you add by 76 from both sides.

`\sf{11x-76+76=7x-44+76}}`

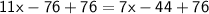
Solve.

`\sf{11x=7x+32}`

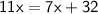
Next, subtract by 7x from both sides.

`\sf{11x-7x=7x+32-7x}`

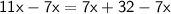
Solve.

`\sf{4x=32}`

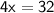
Then, you divide by 4 from both sides.

`\sf{(4x)/(4)=(32)/(4)}`

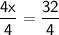
Solve.

Divide these numbers from left to right.

32/4=8

`\rightarrow \boxed{\sf{x=8}}`

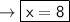
In order for the parallelogram to be a rhombus, x=8.

Therefore, the final answer is x=8.

I hope this helps, let me know if you have any questions.