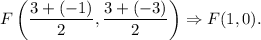Question

What are the coordinates of the midsegment that is parallel to side BC.

(0, 2) and (1, 0)


(0, 2) and (-2, -1)


(1, 0) and (2, 1)


(1, 0) and (-2, -1)

Answer 1

D: (1,0) and (-2,-1)

Explanation:

if you where to plot all the other answers and take a straight edge (ruler) and drew a line through them you would find this is the only proper answer. and i used geogebra

Answer 2

Correct choice is D (1,0) and (-2,-1)

Explanation:

Triangle DBC has vertices B(-3,1), C(3,3) and D(-1,-3). By the triangle midline theorem, the midline joining the midpoints of two sides is parallel to the third side. Thus, you have to find the coordinates of the midpoints of the sides DB and DC of the triangle DBC.

Let point E be the midpoint of the side BD, then point E has coordinates
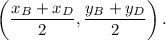`\left((x_B+x_D)/(2),(y_B+y_D)/(2)\right).` If B(-3,1) and D(-1,-3), then

`E\left((-3+(-1))/(2),(1+(-3))/(2)\right)\Rightarrow E(-2,-1).`

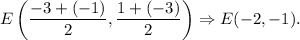
Let point F be the midpoint of the side DC, then point F has coordinates
`\left((x_C+x_D)/(2),(y_C+y_D)/(2)\right).` If C(3,3) and D(-1,-3), then

`F\left((3+(-1))/(2),(3+(-3))/(2)\right)\Rightarrow F(1,0).`