Question

What are the solutions to the equation?

x2 + 6x = 40

x = −10 and x = 4
x = −8 and x = 5
x = −5 and x = 8
x = −4 and x = 10

Answer 1

x = −10 and x = 4

Explanation:

We are given the following quadratic equation and we are to solve it to find the two solution for the variable x:

`x^2+6x=40`

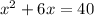
Rearranging the equation by putting the constant on the same side as the variables to get:

`x^(2) +6x-40=0`

Now factorizing it to get:

`x^(2) -4x+10x-40=0\\\\x(x-4)+10(x-4)=0\\\\(x+10)(x-4)=0\\\\x= -10, x= 4`

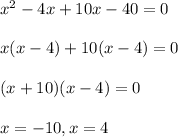
Therefore, the solution to the given quadratic equation
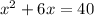`x^2+6x=40` are x = −10 and x = 4.

Answer 2

x = −10 and x = 4

Explanation:

x2 + 6x = 40

Subtract 40 from each side

x^2 + 6x -40 =0

Factor, what 2 numbers multiply to -40 and add to 6

10 * -4 = -40 10+-4 = 6

(x+10) (x-4) = 0

Using the zero product property

x+10 =0 x-4=0

x=-10 x=4