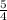Question

If a vertical line is dropped from the x-axis to the point (12, –9) in the diagram below, what is the value of sec?

Answer 1

Answer:C on edge

Explanation:

Answer 2

Value of
`\sec \theta = (5)/(4)`

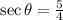
Explanation:

Given: A vertical line is dropped from the x-axis to the point (12, -9) as shown in the diagram below;

To find the value of
`\sec \theta`.

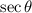By definition of secants;

`\sec \theta = (1)/(\cos \theta)`

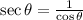
Now, first find the cosine of angle
`\theta`

As the point (12 , -9) lies in the IV quadrant , where
`\cos \theta > 0`

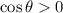
Consider a right angle triangle;

here, Adjacent side = 12 units and Opposite side = -9 units

Using Pythagoras theorem;

`(Hypotenuse side)^2= (12)^2 + (-9)^2 = 144 + 81 = 225`

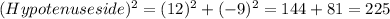
or

`Hypotenuse = √(225) =15 units`

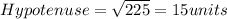
Cosine ratio is defined as in a right angle triangle, the ratio of adjacent side to hypotenuse side.

`\cos \theta = (Adjacent side)/(Hypotenuse side)`

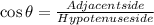
then;

`\cos \theta = (12)/(15) = (4)/(5)`

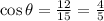
and

`\sec \theta = (1)/(\cos \theta)= (1)/((4)/(5)) = (5)/(4)`

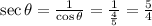
therefore, the value of
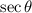`\sec \theta` is,
`(5)/(4)`