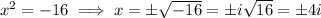Question

Find all the zeros of the equation

-3x^4+27^2+1200=0
if you could show yourworkthat would be great :3

Answer 1

Divide both sides by -3, and replace
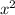`x^2` with
`y`. Then

`-3x^4+27x^2+1200=0\iff y^2-9y-400=0`

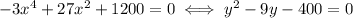
Factorize the quadratic in
`y` to get

`y^2-9y-400=(y+16)(y-25)=0\implies y=-16,y=25`

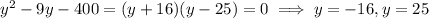
which in turn means

`x^2=-16,x^2=25`

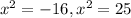
But
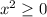`x^2\ge0` for all real
`x`, so we can ignore the first solution. This leaves us with

`x^2=25\implies x=\pm√(25)=\pm5`

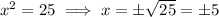
If we allow for any complex solution, then we can continue with the solution we ignored:

`x^2=-16\implies x=\pm√(-16)=\pm i√(16)=\pm4i`