Both 25x and 22x + 9 have the same answers. So you set those two equal to each other.
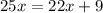
Subtract 22x from both sides.
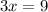
and you're left with this.
Next, divide 3 from both sides.
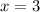
re-insert 3 into one of the equations.
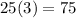
add up both angles
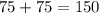
all angles (for quadrilaterals)added up equals 360.
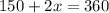
solve for x. Subtract 150 from both sides.
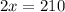
and divide 2 from both sides
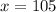
AngleLMN= 105