Answer: The correct option is (A) 30.
Step-by-step explanation: We are given to find the value of x from the figure shown with two intersecting lines.
As noted from the figure, since the measures of two vertically opposite angles are equal, so
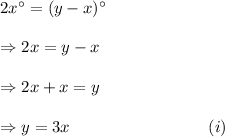
And, from the measure of a straight angle, we can write
![2x^\circ+(y+x)^\circ=180^\circ\\\\\Rightarrow 2x+(y+x)=180\\\\\Rightarrow 3x+y=180\\\\\Rightarrow 3x+3x=180~~~~~~~~~~~~\textup{[putting the value of y from equation (i)]}\\\\\Rightarrow 6x=180\\\\\Rightarrow x=30.](https://img.qammunity.org/2019/formulas/mathematics/middle-school/jwoozizn4cjlptk9sfwwmo6tgdtu2rqmvq.png)
Thus, the required value of x is 30.
Option (A) is CORRECT.