Question

A vector A~ has a negative x component 2.89 units in length and a positive y component 3.17 units in length. The vector A~ can be written in unit vector notation: a1ˆı + a2ˆ. Find the value of (a) a1 (units) (b) a2 (units) (c) What is the magnitude of A~ (units)? (d) What is the direction of A~ (degrees)? 1

Answer 1

a1 = -2.89 units

a2 = 3.17 units

magnitude = 4.2896 units

direction = 132.35 degrees

Step-by-step explanation:

We can write the vector in component form as: <-2.89, 3.17>

That means that its i and j components are:

a1 = -2.89 units

a2 = 3.17 units

The magnitude of the vector is given by:

`√((-2.89)^2 +3.17^2) \approx 4.2896`

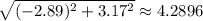
The vectors direction can be found from the tangent function, ad noticing that the vector must reside on the Second Quadrant:

`arctan(-3.17/2.89) + 180^o\approx 132.35^o`