Question

A ball is thrown into the air from a height of 6 feet at time t = 0. The function that models this situation is h(t) = -16t2 + 95t + 6, where t is measured in seconds and h is the height in feet. What is the height of the ball after 2 seconds? What is the maximum height of the ball? When will the ball hit the ground? What domain makes sense for the function? You must show your work on parts a-c to receive credit.

Answer 1

the height of the ball after 2 seconds is 132 feet

Maximum height of the ball is 147.016 feet

domain is t>=0

Explanation:

The function that models this situation is
`h(t) = -16t^2 + 95t + 6`

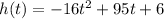
To find the height of the ball after 2 seconds, we plug in 2 for t

`h(2) = -16(2)^2 + 95(2) + 6=132`

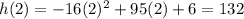
the height of the ball after 2 seconds is 132 feet

To find maximum height we find out vertex

We apply formula x=-b/2a

a=-16 , b= 95

`x=(-b)/(2a) =(-95)/(2(-16))=2.969`

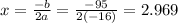
now we plug in 2.969 and find out y

`h=-16(2.969)^2 + 95(2.969) + 6=147.016`

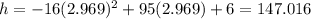
Maximum height of the ball is 147.016 feet

Domain is the set of 't' values for which function is defined

Time 't' cannot be negative so t is all positive real number

domain is t>=0