Answer:
a.
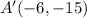
b.
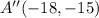
Explanation:
The given point is
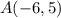 .
.
We want to find the image after this point has been reflected in the line
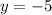 .
.
Recall that, the mapping for a reflection in the line
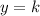 is
is
 .
.
This implies that,
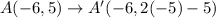 .
.
This simplifies to,
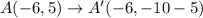 .
.
This will finally give us,
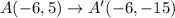 .
.
b. We now apply the second reflection, which is a reflection in the line
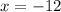 .
.
Recall again that, the reflection in the line
 has the mapping
has the mapping
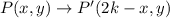 .
.
This implies that,
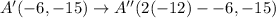 .
.
This implies that,
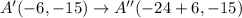 .
.
This will finally give us,
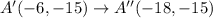 .
.
See graph in attachment.