Question

A piece of wire is 76cm long. It is cut into two unequal parts and each part is bent into a square. The sum of the areas of the two squares is 205cm². Find the length of the shorter part of the wire.

Answer 1

The length of the shorter part of the wire is 24 centimeters.

Explanation:

Let
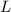`L` the total length of the piece of wire, where
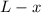`L-x` and
`x` are the perimeters of the greater and lesser squares. All lengths are measured in centimeters. Since squares have four sides of equal length, the side lengths for the greater and lesser squares are
`(L-x)/(4)` and
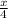`(x)/(4)`. From statement we find that the sum of the areas of the two squares (
`A`), measured in square centimeters, is represented by the following expression:

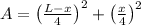`A = \left((L-x)/(4) \right)^(2)+\left((x)/(4) \right)^(2)` (1)

And we expand this polynomial below:

`A = (L^(2)-2\cdot L\cdot x +x^(2))/(16) + (x^(2))/(16)`

`A = (L^(2)-2\cdot L\cdot x +2\cdot x^(2))/(16)`

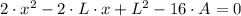`2\cdot x^(2)-2\cdot L\cdot x +L^(2)-16\cdot A = 0` (2)

If we know that
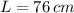`L = 76\,cm` and
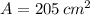`A = 205\,cm^(2 )`, then the length of the shorter part of the wire is:

`2\cdot x^(2)-152\cdot x +2496 = 0`

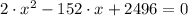
By the Quadratic Formula, we determine the roots associated with the polynomial:

`x_(1) = 52\,cm`,
`x_(2) = 24\,cm`

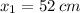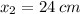
The length of the shorter part of the wire corresponds to the second root. Hence, the length of the shorter part of the wire is 24 centimeters.