Given:
Equation of a line is

To find:
The slope of the line perpendicular to the given line.
Solution:
The slope of the equation
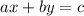 is
is
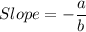
We have,

Here, a=1, b=-3. So, slope of this line is
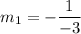

Product of slopes of two perpendicular lines is -1.
Let slope of perpendicular line is
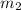 .
.

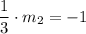
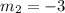
Therefore, the slope of the perpendicular line is -3.