Hello there!
Question:-

We need to find the value of x.
Solution:-

Distribute:-
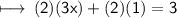
On Simplification:-
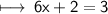
Subtract 2 from both sides :-
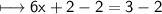
On Simplification:-
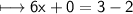
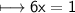
Divide the two sides by 6:-

Cancel 6/6, leave x, answer is 1x. 1/6 can't be cancelled.
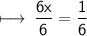
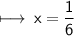
In Decimal:-
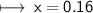
______________________________________
Henceforth,the value of x is :-
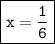

_____________________________________
Please let me know if you have any questions.
~MisterBrian