Question

Chapman’s brickyard sells bricks and blocks. A brick costs $0.38 and a block costs $1.56. The brickyard filled a $24.80 order, which contained 28 items

a. Write a system of equations that can be used to find the number of bricks and blocks in the order.

b. How many blocks were in the order?

Answer 1

(a) A system of equations:

`0.38x+1.56y = 24.80`

`x+y = 28`

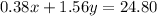
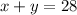
(b) Number of blocks were in the order is, 12

Explanation:

(a)

Let x represents the number of bricks and y represents the number of blocks.

As per the given statement:

A bricks costs $ 0.38 and a block costs $1.56.

Total bricks costs = 0.38x and total block costs = 1.56y

The brickyard filled $24.80 order.

⇒
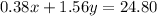`0.38x+1.56y = 24.80` ......[1]

Since, it contained 28 items.

⇒
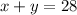`x+y = 28` ....[2]

Multiply equation [2] both sides by 0.38 we have;

`0.38(x+y) = 0.38 * 28`

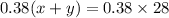
Using distributive property:
`a\cdot (b+c) = a\cdot b + a\cdot c`

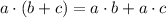
0.38x +0.38 y = 10.64 ......[3]

Subtract equation [3] from [1] to get x eliminated ;

`0.38x+1.56y -0.38x -0.38y= 24.80 - 10.64`

Combine like terms;

1.18y =14.16

Divide both sides by 1.18 we have;

`(1.18y)/(1.18) = (14.16)/(1.18)`

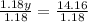
Simplify:

`y = 12`

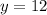
Substitutes this y value in equation [2] to solve for x;

x + 12= 28

Subtract 12 from both sides we get;

x +12-12 =28-12

Simplify:

x = 16

Therefore, a system of equations that can be used to find the number of bricks and the number of blocks in the order:

`0.38x+1.56y = 24.80`

`x+y = 28`

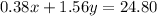
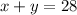
(b)

The number of blocks are in order(y), is 12.