Question

Write the quadratic equation whose roots are 6 and -5, and whose leading coefficient is 3

Answer 1

`y= 3x^2-3x-90`

Explanation:

Intercepts form of quadratic equation is

y= a(x-p) (x-q) where 'a' is the leading coefficient

p and q are the roots

Given that 6 and -5 are the roots

so p = 6 and q= -5

Leading coefficient a= 3

Plug in all the values in the equation

y= a(x-p) (x-q)

y= 3(x-6) (x-(-5))

y= 3(x-6) (x+5)

Now we multiply the parenthesis

`y= 3(x^2+5x-6x-30)`

`y= 3(x^2-x-30)`

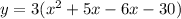
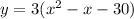
Multiply 3 inside the parenthesis

`y= 3x^2-3x-90`