Answer:
The correct answer option is:
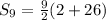
Explanation:
We know that,
the sum of the first
 terms of an Arithmetic Sequence is given by:
terms of an Arithmetic Sequence is given by:
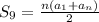
where
 is the number of terms,
is the number of terms,
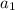 is the first term of the sequence; and
is the first term of the sequence; and
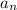 is the first term of the sequence.
is the first term of the sequence.
So for
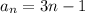 ,
,
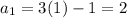
and

Putting these values in the formula to get:
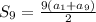
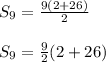
First five terms:
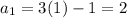
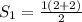 =2
=2
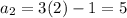
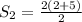 =7
=7
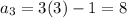
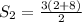 =15
=15
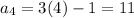
 =26
=26
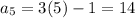
 =40
=40