Question

What is the domain of the relation graphed below?

domain: {–5, –4, –3, –2, 0, 1, 2, 3, 4, 5}
domain: {–3, –2, 0, 1, 4}
domain: {–5, –4, –3, 1, 2, 5}
domain: {(–5, 0), (–4, 1), (–3, 4), (1, –2), (2, 4), (5, –3)}

Answer 1

The correct option is 3.

Explanation:

The set of inputs is called domain and the set of outputs is called range.

If a relation is defined as

`R=\(x,y)`

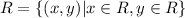
Then the domain of the relation is
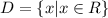`D=\x\in R\` and range of the relation is
`R=\y`

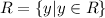
From the given graph it is clear that the coordinate pairs of the relation are (-5,0), (-4,1), (-3,4), (1,-2), (2,4),(5,-3).

The relation defined by the graph is

R = {(-5,0), (-4,1), (-3,4), (1,-2), (2,4),(5,-3)}

The domain of the relation is

Domain: {–5, –4, –3, 1, 2, 5}

Therefore the correct option is 3.

Answer 2

C) -5, -4, -3, 1, 2, 5

Explanation:

Remember domain is the x and range is the y. So, to find the domain you have to get all the x-values in the graph, not the y-values. Another thing to make sure is to list them from least to greatest. Otherwise, your answer may get counted as wrong. Hope I helped! :)