Question

Rajan needs 1 liter of 15% hydrochloric acid solution for an experiment. He checks his storage cabinet, but he only has a 5-liter bottle of 5% hydrochloric acid solution and a 1-liter bottle of 45% hydrochloric acid solution. How many liters of the 5% hydrochloric acid and the 45% hydrochloric acid solutions should Rajan combine to make 1 liter of a solution that is 15% hydrochloric acid?

Answer 1

Rajan must use 0.75 liters of 5% hydrochloric acid solution and 0.25 liters of 45% hydrochloric acid solution.

Explanation:

Let us assume that, x liters of the 5% hydrochloric acid and y liters of the 45% hydrochloric acid solutions are combined.

As Rajan need total of 1 liter of solution, so

`x+y=1`

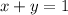
i.e
`x=1-y` --------------------1

As Rajan needs 5% hydrochloric acid and 45% hydrochloric acid to make a 1 liter batch of 15% hydrochloric acid, hence acid content of the mixture of two acids will be same as of the final one, so

`0.05x+0.45y=1* 0.15`

i.e
`5x+45y=15` -------------2

Putting value of x from equation 1 in equation 2,

`\Rightarrow 5(1-y)+45y=15`

`\Rightarrow 5-5y+45y=15`

`\Rightarrow 5+40y=15`

`\Rightarrow 40y=15-5=10`

`\Rightarrow y=0.25`

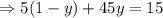
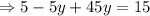
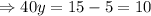
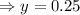
Putting the value of y in equation 1,

`x=1-0.25=0.75`

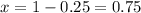
Therefore, Rajan must use 0.75 liters of 5% hydrochloric acid solution and 0.25 liters of 45% hydrochloric acid solution.