Question

One x-intercept of the graph of the of the cubic function f(x) = x^3 – 2x^2 – 111x – 108 is –9.

What are the other zeros?

Answer 1

The zeros of the given function are -9,-1 and 12.

Explanation:

The given function is

`f(x)=x^3-2x^2-111x-108`

It is given that the x-intercept of the graph is -9, therefore -9 is a zero of the function.

Since -9 is the zero of the function, therefore (x+9) is a factor of f(x). Use synthetics method or long division method to divide the function by (x+9).

`f(x)=(x+9)(x^2-11x-12)=0`

`f(x)=(x+9)(x^2-12x+x-12)=0`

`f(x)=(x+9)(x(x-12)+(x-12))=0`

`f(x)=(x+9)(x-12)(x+1)=0`

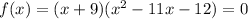
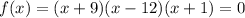
Use zero product property and quote each factor equal to 0.

`x=-9,-1,12`

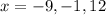
Therefore zeros of the given function are -9,-1 and 12.