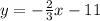Answer:
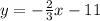
Explanation:
Th equation of line is given by:

where, m is the slope and b is the y-intercept.
As per the statement:
Given the point slope form equation:
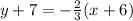
Using distributive property,
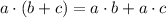
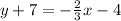
subtract 7 from both sides we have;
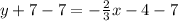
Simplify:
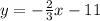
Therefore, a linear function represents the line given by the point-slope equation is,