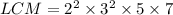ANSWER
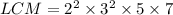
EXPLANATION
The prime factorization of the two numbers are
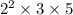
and
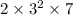
To find the least common multiple , we collect the factors with the powers and multiply them out.
The highest power of 2 is
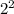
The highest power of 3 is
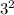
The highest power of 5 is
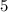
and the highest power of 7 is
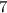
Therefore