Question

Perfect patios is building a rectangular deck for a customer. According to the customer's specifications ,the perimeter should be 40 meters and the difference between twice the length and twice the width should be 4 meters . I is the length and w is the width.What will be the length and width of the deck?

Answer 1

The length and width of the deck will be 11 meters and 9 meters respectively.

Explanation:

The length of the rectangular deck is
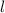`l` meter and width is
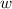`w` meter.

The perimeter of the deck should be 40 meters. So, the first equation will be...

`2(l+w)= 40\\ \\ \Rightarrow l+w = 20 ..................................(1)`

The difference between twice the length and twice the width should be 4 meters. So, the second equation will be....

`2l-2w= 4\\ \\ \Rightarrow l-w= 2 .................................(2)`

Adding equation (1) and (2), we will get.....

`(l+w)+(l-w)= 20+2\\ \\ 2l= 22\\ \\ l= (22)/(2)= 11`

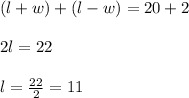
Now plugging this
`l=11` into equation (1), we will get....

`11+w= 20\\ \\ \Rightarrow w=20-11= 9`

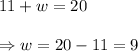
So, the length and width of the deck will be 11 meters and 9 meters respectively.