Question

How many solutions can be found for the system of linear equations represented on the graph?

A) no solution
B) one solution
C) two solutions
D) infinitely many solutions

Answer 1

Answer: A) no solution

Explanation:

The equation of first line =
`y=-(1)/(2)x+4`

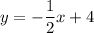
which can be written as :
`2y=-x+8`

`x+2y=8`

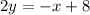
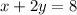
The equation of second line =
`y=-(1)/(2)x-6`

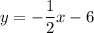
which can be written as :
`x+2y=-12`

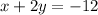
Since , the ratio of the coefficients of x is equal to the ratio of the coefficients of y but not the ratio of the constants .

`(1)/(1)=(2)/(2)\\eq(8)/(-12)`

Therefore , the lines are parallel and do not have any solution.

So , the correct answer is A) no solution.

Answer 2

A) no solution

Explanation:

These lines are parallel. This means they never intersect; since the solution to a system of equations is the point or points of intersection, this means there is no solution to this system.