Answer:
Length a = 59.62
Width b = 67.09
Cost = $10,734.4
Explanation:
Solution:
Let a be the length and b be the width.
Area of the rectangle = a x b
a x b = 4000
Cost of the construction:
Cost = 100a + 80(a + 2b)
Cost = 100a + 80a + 160b
Cost = 180a + 160b Equation of the Cost of the construction.
Now, we need a and b to calculate the minimum cost required to build a lot.
From the area = a x b
we have,
a x b = 4000
b = 4000/a
Putting this equation into the cost of the construction.
We get:
Cost = 180a + 160 x (4000/a)
Cost = 180a + 640000/a
Differentiating with respect to a, we get
 = 180 - 640000/
= 180 - 640000/
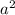
Putting
 = 0
= 0
180 - 640000/
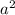 = 0
= 0
Taking LCM
180
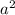 - 640000 = 0
- 640000 = 0
Solving for a
180
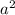 = 640000
= 640000
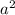 = 640000/180
= 640000/180
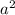 = 3555.55
= 3555.55
Taking square root
a = 59.62
As we know,
b = 4000/a
putting the minimum value of a = 59.62
b = 4000/59.62
b = 67.09
So, now we have both the dimensions a and b
Putting the values of a and b we will get the cost of the construction.
Cost = 180a + 160b
Cost = 180(59.62) + 160(67.09)
Cost = $10,734.4
Hence, $10,734.4 will be the minimum cost of the construction for Bill Ding.