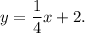Question

The point-slope form of the equation of a line that passes through points (8, 4) and (0, 2) is y – 4 = (x – 8). What is the slope-intercept form of the equation for this line?

Answer 1

y = -4x-8

Explanation:

-4 is your slope and -8 is your y-intercept. The standard slope intercept formula is y = mx+b where m is your slope, b is your y-intercept, and x and y are x and y. All you have to do is plug in your variables and you are left with y = -4x-8

Answer 2

Answer: The required slope-intercept form of the given line is

`y=(1)/(4)x+2.`

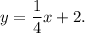
Step-by-step explanation: Given that the point-slope form of the equation of a line that passes through points (8, 4) and (0, 2) is as follows :

`y-4=(1)/(4)(x-8)~~~~~~~~~~~~~~~~~~~~~~~~~~~(i)`

We are to find the slope-intercept form of the equation (i).

We know that

the slope-intercept form of the equation of a straight line with slope m and y-intercept c is given by

`y=mx+c.`

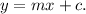
From equation (i), we have

`y-4=(1)/(4)(x-8)\\\\\\\Rightarrow y-4=(1)/(4)x-2\\\\\\\Rightarrow y=(1)/(4)x-2+4\\\\\\\Rightarrow y=(1)/(4)x+2.`

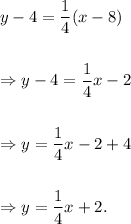
Thus, the required slope-intercept form of the given line is

`y=(1)/(4)x+2.`