Question

The data values in the normal distribution curve have a mean of 112 with a standard deviation of 19.2. Find the area of the shaded region.

Please help if you could explain it as well that’s be great

Answer 1

0.8154

Explanation:

sorry I dont have an explanation but I know it's right. I just took the test

Answer 2

0.8154

Explanation:

The data values in the normal distribution curve have a mean of 112 with a standard deviation of 19.2

Probability of shaded region is P(94<=x<=156)

P(94<=x<=156) = P(x<156) - P(x<94)

We use formula

`z=(x-mean)/(standard deviation)`

`z=(156-112)/(19.2)=2.29`

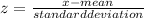
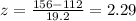
z= 2.29 (Use z-score table to find value)

z-score value is 0.9890

So P(x<156)= 0.9690

Now we find P(x<94)

`z=(94-112)/(19.2)=-0.94`

z= -0.94 (Use z-score table to find value)

z-score value is 0.1736

So P(x<94)= 0.1736

P(94<=x<=156) = P(x<156) - P(x<94)

P(94<=x<=156)= 0.9690 - 0.1736= 0.8154