Question

What is the common ratio for the geometric sequence? 18,12,8,163,… Enter your answer in the box.

Answer 1

2/3

I took test and got this answer confirmed

Answer 2

`(2)/(3)`.

Step-by-step explanation:

We have been given a geometric sequence 18,12,8,16/3,.. We are asked to find the common ratio of given geometric sequence.

We can find common ratio of geometric sequence by dividing any number by its previous number in the sequence.

`\text{Common ratio of geometric sequence}=(a_2)/(a_1)`

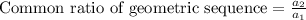
Let us use two consecutive numbers of our sequence in above formula.

`a_2` will be 12 and
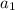`a_1` will be 18 for our given sequence.

`\text{Common ratio of geometric sequence}=(12)/(18)`

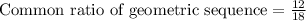
Dividing our numerator and denominator by 6 we will get,

`\text{Common ratio of geometric sequence}=(2)/(3)`

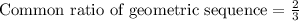
Let us use numbers 8 and 16/3 in above formula.

`\text{Common ratio of geometric sequence}=((16)/(3))/(8)`

`\text{Common ratio of geometric sequence}=(16)/(3*8)`

`\text{Common ratio of geometric sequence}=(2)/(3)`

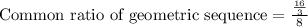
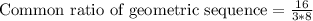
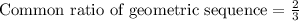
Therefore, we get
`(2)/(3)` as common ratio of our given geometric sequence.