Answer:
0.82% probability that the average time it takes to complete both homework assignments is greater than 82 minutes
Explanation:
When the distribution is normal, we use the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
Math homework:
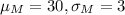
Arts homework:
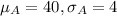
What is the probability that the average time it takes to complete both homework assignments is greater than 82 minutes?
Here, we have a sum of normal variables. The mean will be the sum of the means, while the standard deviation is the square root of the sum of the variances. So
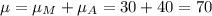
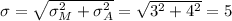
This probability is 1 subtracted by the pvalue of Z when X = 82. So

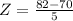
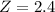
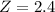 has a pvalue of 0.9918
has a pvalue of 0.9918
1 - 0.9918 = 0.0082
0.82% probability that the average time it takes to complete both homework assignments is greater than 82 minutes