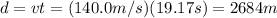Question

Alex, a rescue pilot drops a survival kit while her plane is flying horizontally at an altitude of

1800.0 m with a forward velocity of 140.0 m/s A group of lost Antarctica explorers is below on the   
            ground.
How far in front of the explorers should Alex drop the supplies?

Answer 1

2684 m

Step-by-step explanation:

First of all, we should find the time that the survival kit takes to reach the ground. For this purpose, we can only consider the vertical motion of the kit, which is an accelerated motion with constant acceleration g=9.8 m/s^2 (toward the ground) and initial vertical velocity of zero. The vertical distance travelled is S=1800.0 m, so we can find the time by using:

`S=(1)/(2)at^2\\t=\sqrt{(2S)/(a)}=\sqrt{(2\cdot 1800 m)/(9.8 m/s^2)}=19.17 s`

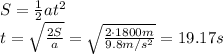
And now we can find the horizontal distance travelled by the kit during this time interval, which corresponds to the distance at which Alex should drop the supplies. The horizontal motion is a uniform motion with constant speed v = 140.0 m/s, so the horizontal distance is

`d=vt=(140.0 m/s)(19.17 s)=2684 m`