Question

Given: rectangle ABCD is similar to rectangle ZBXY. If ZY = 5, XC = 3, DC = 4, then XY =

Answer 1

The answer is 2 1/2 I just did the assignment and that was the answer.

Explanation:

Answer 2

The length of XY is either 10 or 2.5.

Explanation:

Given information: ZY = 5, XC = 3 and DC = 4.

Case 1: Rectangle ABCD is smaller than ZBXY.

The opposite sides of the rectangle are same.

`ZY=BC+XC`

`5=BC+3`

`2=BC`

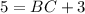
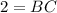
The length of ABCD is 4 and width of ABCD is 2. The width of ZBXY is 5. The corresponding sides of the similar rectangles are is in the same proportion.

`(4)/(XY)=(2)/(5)`

`(4* 5)/(2)=XY`

`10=XY`

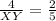
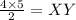
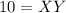
Therefore the value of XY is 10.

Case 2: Rectangle ABCD is larger than ZBXY.

`ZY=BC-XC`

`5=BC-3`

`8=BC`

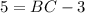
The length of ABCD is 4 and width of ABCD is 8. The width of ZBXY is 5. The corresponding sides of the similar rectangles are is in the same proportion.

`(4)/(XY)=(8)/(5)`

`(4* 5)/(8)=XY`

`2.5=XY`

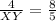
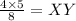
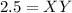
Therefore the value of XY is 2.5.