Question

A manufacturer of banana chips would like to know whether its bag filling machine works correctly at the 449.0 gram setting. It is believed that the machine is underfilling the bags. A 36 bag sample had a mean of 444.0 grams. A level of significance of 0.1 will be used. Determine the decision rule. Assume the standard deviation is known to be 22.0. Enter the decision rule.

Answer 1

|Z| = |-1.36| < 1.645 at 0.1 level of significance

The null hypothesis is accepted

A manufacturer of banana chips are filling machine works correctly at the mean 449

Explanation:

Step(i):-

Given mean of the Population(μ) = 449

The Standard deviation of the population(σ) = 22

size of the sample 'n' = 36

mean of the sample(x⁻) = 444

Given the level of significance(α) = 0.1

Critical value Z = 1.645

Step(ii):-

Null hypothesis:H₀:

A manufacturer of banana chips would like to know whether its bag filling machine works correctly at the 449.0

(μ) = 449

Alternative Hypothesis:H₁: (μ) ≠ 449

Test statistic

`Z = (x^(-) -mean)/((S.D)/(√(n) ) ) \\= (444-449)/((22)/(√(36) ) )`

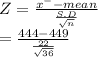
= -1.36

Final answer:-

|Z| = |-1.36| < 1.645 at 0.1 level of significance

The null hypothesis is accepted

A manufacturer of banana chips are filling machine works correctly at the mean 449