Question

What is the length of BD?

What is the length of segment AC?
What is the area of the triangle ABC?

Answer 1

BD = 17.32 units

AC = 20 units

Area of triangle ABC = 173.2 square units

Explanation:

BD is the height of triangle BAD.

we can calculate it's length by using trigonometric knowledge: sin 60° = opposite/hypotenuse

Sin 60° = BD/20

BD = 20 Sin 60°

BD = 17.32 units

Since angle BAD = Angle BCD. this means that line BA = line BC

we therefore can conclude that BD divides AC into two equal parts

Hence Cos 60° = AD/BA

Cos 60° = AD/20

AD = 20 Cos 60°

AD = 10 units

AC = 10x 2

= 20 units

area of triangle ABC = Area of triangle 2(ABD)

= 2 x 1/2bh

= 2 x 1/2 x 10 x 17.32

= 173.2 square units

Answer 2

BD = 10√3 units

AC = 20 units

Area ΔABC = 100√3 units²

Explanation:

Interior angles of a triangle sum to 180°:

`\implies \angle A + \angle B + \angle C =180^(\circ)`

`\implies 60^(\circ) + \angle B +60^(\circ) =180^(\circ)`

`\implies \angle B +120^(\circ) =180^(\circ)`

`\implies \angle B +120^(\circ) -120^(\circ)=180^(\circ)-120^(\circ)`

`\implies \angle B =60^(\circ)`

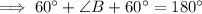
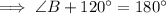
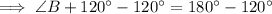
As the interior angles of an equilateral triangle are congruent, and ∠A=∠B=∠C then ΔABC is an equilateral triangle.

In an equilateral triangle, all three sides have the same length.

`\implies AC=BC=AB=20\; \sf units`

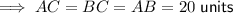
Height of an equilateral triangle:

`\boxed{\textsf{h}=(√(3))/(2)a}`

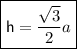
Where a is the side length of the triangle.

As BD is perpendicular to AC, BD is the height of the triangle.

`\begin{aligned}\implies BD & = (√(3))/(2)(20)\\ & =10√(3)\; \sf units \end{aligned}`

Area of an equilateral triangle:

`\boxed{\textsf{A}=(√(3))/(4)a^2}`

Where a is the side length of the triangle.

Therefore:

`\begin{aligned}\implies \textsf{Area of $\triangle ABC$} & =(√(3))/(4)(20)^2\\& = (√(3))/(4)(400)\\& = 100√(3)\; \sf units^2\end{aligned}`