Question

A stuntman drives a car with a mass of 1500 kg on a drawbridge. The car accelerates with a constant force of 10,000 N. While he is driving, the drawbridge is raised to an incline of 30°. What is the car's new acceleration on this incline, ignoring the force due to air resistance? (recall that g=9.8 m/s^2)

Answer 1

1.77 m/s^2

Step-by-step explanation:

There are two forces acting on the car along the direction parallel to the incline:

- The driving force of 10,000 N, which pushes forward

- The component of the weigth of the car parallel to the incline, which pulls backward

The component of the weight of the car parallel to the incline is:

`W_p = mg sin \theta=(1500 kg)(9.8 m/s^2)( sin 30^(\circ))=7350 N`

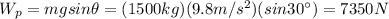
So now we can apply Newton's second law to find the acceleration of the car:

`F-W_p = ma\\a=(F-W_p)/(m)=(10000 N-7350 N)/(1500 kg)=1.77 m/s^2`