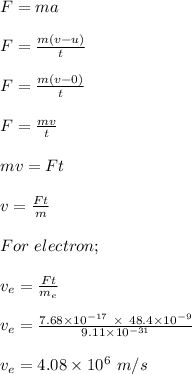Answer:
- The speed of electron is 4.08 x 10⁶ m/s
- The speed of proton is 2,225.82 m/s
Step-by-step explanation:
Given;
electric field, E = 480 N/C
mass of electron, Me = 9.11 x 10⁻³¹ kg
mass of proton, Mp = 1.67 x 10⁻²⁷ kg
time of motion, t = 48.4 ns = 48.4 x 10⁻⁹ s
initial velocity of the particles, u = 0 (initially at rest)
let the speed of each particle after 48.4 ns = v
the magnitude of charge of the particles, q = 1.6 x 10⁻¹⁹ C
The force experienced by each particle is calculated as;
F = Eq
F = (480 N/C) x (1.6 x 10⁻¹⁹ C)
F = 7.68 x 10⁻¹⁷ N
The speed of each particle after 48.4 ns is calculated as;