Answer:
Explanation:
Given :
20 matches were played in a small chess tournament.
Each participant played 2 games with every other participant in the tournament.
To Find : how many people were involved?
Solution :
Let no. of players involved be n
Since we know that for every match there should be two players out of n
So, number of ways they can play :
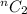
We are also given that each participant played 2 games with every other participant.
So, total no. of games played =
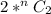
Since we are given that total no. games played = 20
⇒
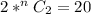
⇒

⇒
 --(a)
--(a)
Formula of combination:
⇒
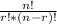
So, solving (a) further using formula
⇒
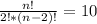
⇒
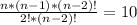
⇒

⇒
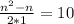
⇒
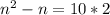
⇒
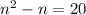
⇒
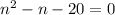
⇒
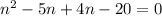
⇒
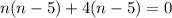
⇒
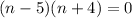
⇒
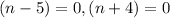
⇒ n = 5 , n =-4
Neglect the negative value since no. of players cannot be negative.
Thus no. of player involved is 5.