Answer:
a.
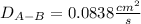
b.

Step-by-step explanation:
Hello!
In this case, since the Hirschfelder's equation is:
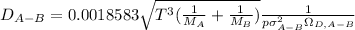
Whereas M is the molar mass and sigma is related to the size of the molecule and omega the collision integral depending on the dimensionless temperature and are parameters related to the Chapman-Enskog theory and the Lenard-Jones parameters which have been tabulated for sulfur dioxide, nitrogen, hydrogen and air. Thus, we proceed as follows:
a. In this case, we have that sigma for sulfur dioxide is 4.026 and that of nitrogen is 3.667, and the parameter e/K is 363 K and 99.8 K respectively.
It means that the pairs are:
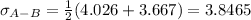

For which:
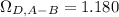
Based on Bird's E2 table.
Now, by plugging in the data, we obtain the following diffusion coefficient:
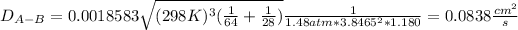
b. In this case, we have that sigma for hydrogen is 2.915 and that of air is 3.617, and the parameter e/K is 30.8 K and 97.0 K respectively.
It means that the pairs are:
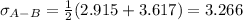
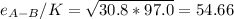
For which:
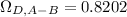
Based on Bird's E2 table.
Now, by plugging in the data, we obtain the following diffusion coefficient:
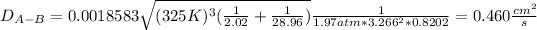
Best regards!