Question

The functions f(x) and g(x) are defined below.

f(x) = -2x -6
g(x) = x^2 + 4x -1

By graphing, determine where f(x) = g(x).
A. x = -3 ; x= -6


B. x= -5 ; x=1


C. x=1 ; x=-4


D. x=-5 x=4

Answer 1

At x=-5 and x=-1 f(x)=g(x)

Explanation:

We have given that :
`f(x) = -2x -6`

`g(x) = x^2 + 4x -1`

To determine : where f(x) = g(x)

Solution : We refer the attached graph which clearly shows that f(x) and g(x) intersect at pt (-5,4) and (-1,-4)

which implies at x= -5 and x=-4 f(x)= g(x)

or At x= -5

`f(x)=-2x-6=-2(-5)-6=4`

`g(x)=x^2+4x -1= (-5)^2+4(-5)-1=4`

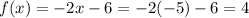
or At x= -1

`f(x)=-2x-6=-2(-1)-6=-4`

`g(x)=x^2+4x -1= (-1)^2+4(-1)-1=-4`

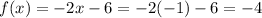
Therefore, at x=-5 and x=-1 f(x)=g(x)

Answer 2

x=-5, x=-1

Explanation:

looking at the graph

x= -5,

x=-1