Answer: Option (A) The new standard deviation is greater than $27.
Step-by-step explanation:
If a sixth skateboard having price of $450 is added to the sample, the new sample set will be the following:
75, 82, 100, 120, 140, 450.
Let us first find the mean of the above sample set.
Mean =
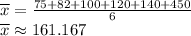
Now that we have mean, let's find the variance.
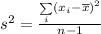
Where n is the number of samples in the set (which in this case is 6).
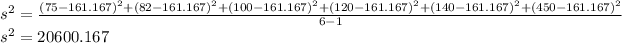
Now that we have variance, it's time to find the new standard deviation by taking the square-root of the variance, as follows:

$143.53 > $27
New standard deviation is greater than the old standard deviation.
Therefore, the correct answer is Option (A) The new standard deviation is greater than $27.