Question

Here are the prices of five new skateboards, in dollars

Answer 1

The correct answer is: Option (A) The new standard deviation is greater than $27.

Step-by-step explanation:

The given sample set is the following:

75, 82, 100, 120, 140.

To find the new standard deviation, add the sixth skateboard to the above sample set, as follows:

75, 82, 100, 120, 140, 450.

Now we have 6 elements in the sample set.

Step-1: Find the mean of the new sample set.

`Mean = \overline{x}=\frac{\underset{i}\sum x_i}{n}`

Where, n is the total number of elements in the sample set. In this case, n=6.

`\overline{x}=(75+82+100+120+140+450)/(6)\\ \overline{x} \approx 161.167`

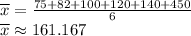
Step-2: Find the variance (
`s^2`).

`s^2 = \frac{\underset{i} \sum (x_i - \overline x)^2}{n-1}`

`s^2 = ((75-161.167)^2+(82-161.167)^2+(100-161.167)^2+(120-161.167)^2+(140-161.167)^2+(450-161.167)^2)/(6-1) \\s^2 = 20600.167`

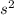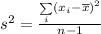
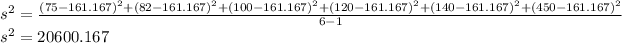
Step-3: Find the new standard deviation.

Standard deviation is the square-root of variance.

`√(s^2) = √(20600.167) \\s \approx 143.53`

New standard deviation ($143.53) is greater than the standard deviation ($27) without the sixth skateboard sample in sample set.

Conclusion: The Option (A) The new standard deviation is greater than $27 is the right answer.