Question

Hat is the slope-intercept form of the function described by this table?

x 1 2 3 4
y 1 −2 −5 −8
Enter your answers in the boxes.
y = x +

Answer 1

Answer: y = -3

x + 4

I just took this quiz and these are the correct answers.

Answer 2

The slope-intercept form of the function described by the table is
`y=-3x+4`

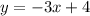
Explanation:

The slope-intercept form for the equation of any straight line is given by:

`y=mx+b`

where:

m is the slope of the line

b is the y-intercept of the line

To find the slope of the line you can choose two points from the table given, for example (2,-2) and (3,-5) and use the fact that the slope is
`m=(y_(2)-y_(1))/(x_(2)-x_(1)) = (-5-(-2))/(3-2)=-3`.

To find the y-intercept b, you can use for example the point (2,-2) or any other point given in the table and use this relation
`b=y_(1)-mx_(1)`,
`b=y_(1)-mx_(1) = -2-((-3)*2)=4`.

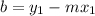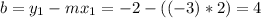So, the slope-intercept form of the function described by the table is
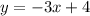`y=-3x+4`.