Question

Determine whether the following function is linear or quadratic.

Identify the quadratic, linear, and constant terms.
Please show all of your work

f(x) = 3x(x-1) - (3x+7)

Answer 1

Linear function:

`f(x)=mx+b`

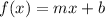
Quadratic function:

`f(x)=ax^2+bx+c`

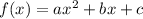
m, a, b, c are any real number.

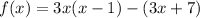`f(x)=3x(x-1)-(3x+7)` use distributive property

`f(x)=(3x)(x)+(3x)(-1)-3x-7=3x^2-3x-3x-7=3x^2-6x-7`

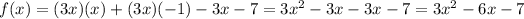
It's a quadratic function.

`f(x)=ax^2+bx+c`

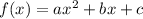
quadratic term: ax²

linear term: bx

constant term: c

Therefore your answer is:

quadratic term = 3x²

linear term = -6x

constant term = -7

Answer 2

Quadratic Function

Explanation:

3x(x-1) -(3x+7)

First, you need to simplify by multiplying what's outside the parenthesis with what's inside.

3x(x) - 3x

3x²-3x = -3x-7

this would become 3x²-6x-7