Question

a 3.0 kg rock is moving at a constand speed in a 0.75 m radius horizontal circle every 2.0 seconds. Calculate the centripetal force acting on the rock

Answer 1

22.3 N

Step-by-step explanation:

The centripetal force acting on a the rock is given by:

`F=m(v^2)/(r)`

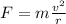
where

m = 3.0 kg is the mass of the rock

v is the speed of the rock

r = 0.75 m is the radius of the trajectory

We need to find the speed of the rock. We know that the rock makes a complete circle in t = 2.0 s, and the radius of the circle is r = 0.75, so the speed is equal to the ratio between the distance covered (the perimeter of the circle) and the time taken:

`v=(2 \pi r)/(t)=(2 \pi (0.75 m))/(2.0 s)=2.36 m/s`

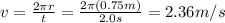
And so, the centripetal force is

`F=m(v^2)/(r)=(3.0 kg)((2.36 m/s)^2)/(0.75 m)=22.3 N`