Question

A guitar string with a length of 80.0 cm is plucked. The speed of a wave in the string is 400 m/sec. Calculate the frequency of the first, second, and third harmonics.

Answer 1

The first harmonic is: 250Hz, second harmonic 500Hz, third harmonic 750Hz.

Step-by-step explanation:

Use the frequency f, speed v, and wavelentgh L relationship:

`v = f\cdot L\implies f = (v)/(L)`

We are given the speed v=400 m/s. The base wavelength on a string of length 80cm is twice the length of the string (a "half wave" along the full length of the string), so:

`f = (400(m)/(s))/(2\cdot0.8 m)= 250(1)/(s)=250 Hz`

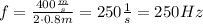
The fundamental frequency (first harmonic) is 250 Hz

The second harmonic is produced by one full wave across the string (adding one node in the middle), so L=80cm in this case, therefore the second harmonic frequency is: f2 = 2*250=500Hz

the third harmonic add another node (and a half wave) to the pattern and the wavelength will be 2/3 of 80cm, so f3=3*250Hz = 750Hz