Question

Which of the following are measurements for quadrilaterals that are similar to a quadrilateral with sides measuring 4, 6, 8, and 10? Check all that apply

Answer 1

Options C, D and E are correct choices.

Explanation:

Since the corresponding sides of similar figures are always proportional, so let us check which of the given measurements have same proportions for all sides to the quadrilateral with sides measuring 4, 6, 8, and 10.

A.
`(4)/(1) =(6)/(2) =(8)/(4) =(10)/(5)`

`4\\eq 2=2=2=2`

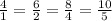
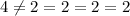
We can see that one side in not proportional to its corresponding, whether other sides are proportional. Therefore, the measurements given in option A are not of a similar quadrilateral to our given quadrilateral with sides measuring 4,6,8 and 10.

B.
`(4)/(6) =(6)/(8) =(8)/(10) =(10)/(12)`

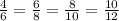
Upon simplifying our proportions we will get,

`(2)/(3)\\eq (3)/(4)\\eq (4)/(5)\\eq (5)/(6)`

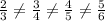
Therefore, the measurements given in option B are not of a similar quadrilateral to our given quadrilateral with sides measuring 4,6,8 and 10.

C.
`(4)/(6) =(6)/(9) =(8)/(12) =(10)/(15)`

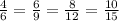
Upon simplifying our proportions we will get,

`(2)/(3)=(2)/(3)=(2)/(3)=(2)/(3)`

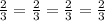
We can see that measurements given for the quadrilateral in option C are proportional to corresponding sides (4,6,8,10) of the quadrilateral , therefore, option C is the correct choice.

D.
`(4)/(2) =(6)/(3) =(8)/(4) =(10)/(5)`

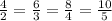
Upon simplifying our proportions we will get,

`2=2=2=2`

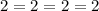
We can see that measurements given for the quadrilateral in option D are proportional to corresponding sides of quadrilateral with sides measuring 4,6,8 and 10, therefore, option D is the correct choice.

E.
`(4)/(12) =(6)/(18) =(8)/(24) =(10)/(30)`

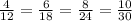
Upon simplifying our proportions we will get,

`(1)/(3)=(1)/(3)=(1)/(3)=(1)/(3)`

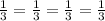
We can see that measurements given for the quadrilateral in option E are proportional to corresponding sides of quadrilateral with sides measuring 4,6,8 and 10, therefore, option E is the correct choice.